Slide rule A simple explanation of a clever calculator

Dear reader, The other day I was talking to a lady who had many mathematical qualifications but had never seen a slide rule. This is a bit like an inter-galactic astronaut admitting they weren't quite sure where Mars was let alone visited it! So here is a little page for all those people who missed out on a very clever and, in its day, essential calculating device. We better start with Logarithms. If you've heard the word and it frightens you - don't worry. They are simple and amazing. Then you can make your own slide rule in two minutes.

Logarithms

A logarithm is a magic number related to another number. Don't worry how you calculate the magic number. In the olden days we just looked up tables printed in books. Today if you need a logarithm your scientific calculator or computer will tell you. Any number: Logarithm:

The magic is that if you add the logarithms from two numbers you get the logarithm of the number you'd otherwise get by multiplying the two original numbers. First number Logarithm Press the + below to calculate Second number Logarithm Logarithms added: which is logarithm of Change the numbers outlined in red and recalculate to see what happens.

Here is a table of logarithms for numbers 1 to 99. Read the tens down the side and the units across the top. 0 1 2 3 4 5 6 7 8 9 0 0 .301 .477 .602 .698 .778 .845 .903 .954 10 1 1.041 1.079 1.113 1.146 1.176 1.204 1.23 1.255 1.278 20 1.301 1.322 1.342 1.361 1.38 1.397 1.414 1.431 1.447 1.462 30 1.477 1.491 1.505 1.518 1.531 1.544 1.556 1.568 1.579 1.591 40 1.602 1.612 1.623 1.633 1.643 1.653 1.662 1.672 1.681 1.69 50 1.698 1.707 1.716 1.724 1.732 1.74 1.748 1.755 1.763 1.77 60 1.778 1.785 1.792 1.799 1.806 1.812 1.819 1.826 1.832 1.838 70 1.845 1.851 1.857 1.863 1.869 1.875 1.88 1.886 1.892 1.897 80 1.903 1.908 1.913 1.919 1.924 1.929 1.934 1.939 1.944 1.949 90 1.954 1.959 1.963 1.968 1.973 1.977 1.982 1.986 1.991 1.995 I have shown how to look up 64 as an example. These tables came in books called "Log tables". To deal with numbers accurate to more decimal places the tables were much larger, with say 1000ths down the side and 10,000ths across the top with a bit of guesswork in-between if you wanted a bit more accuracy. Do you notice that the log of 64 is twice the log of 8? What do you notice about 3 and 30, 4 and 40 and so on?

But what about all those numbers from minus-as-much-as-you-want to plus as-much-as-you-want? Surely you would need an enormous book to deal with 4, 40, 4 thousand, 4 million and so on? Lets see some more logarithmic cleverness: Number Logarithm 4 0.6021 40 1.6021 400 2.6021 4000 3.6021 40000 4.6021 See what's happening? If the logarithm of 10 is 1 then to multiply by ten add 1. (To divide, subtract). This means that a book of log tables only needed to cover 0.0001 to 0.9999 in 1000 lines of ten columns to be accurate to four decimal places.

The logarithm of 24 is the logarithm of 2.4(0.38) plus 1 = (1.38) Here is an example: Item Number Tens Normalised Look-up log Full log Hours in a day 24 1 2.4 0.3802 1.3802 Days in a week 7 0 7.0 0.8451 0.8451 Weeks in a year 52 1 5.2 0.7160 1.7160 Years in a century 100 2 1.0 0.0000 2.0000 X X + Hours in a century 873600 5 8.7 0.9413 5.9413 Divide Number by ten, (repeating if necessary) until it is less than 10. The Tens column is how many times we had to do this. The Normalised column is the same number with the decimal point shifted Tens times. The Look-up log is the logarithm of the Normalised number as we might find by looking in a book of log tables. The Full log is the Look-up log plus the Tens column. The yellow column adds up exactly, but there is something going on with the other columns. What has happened is that the light blue column has done a 'carry one' (times ten). If you multiply the pink colum items you get 87.36 (times ten). The ochre column adds to 4 not 5. (Times ten again). All these are the same thing it is just that we have had to scale down by an extra decimal place.

The slide rule

Now you know how logarithms work you may be wondering if you can have a go. Actually to sit down with a book of log tables is a little bit more complicated than I have explained so far. For now let's stick with the rough and ready explanation above. (You can find out about division and fractions later.) But now let us look at the very clever pocket calculator called the slide rule. If I want to multiply 2 by 3 I have to add the logs of these together. I could get my pencil and paper and log books out and do the sum log of 2 ( = 0.301) plus log of 3 ( = .4771) added together is .7782 which by looking in my tables is the log of 6. But suppose I had measured a line 3.01 centimetres and added another line onto the end of it 4.771 centimetres long. Now I have a line 7.782 centimetres long. If only I had a clever way of measuring out these distances... ... Here's how it's done. Print out this page then cut out the picture below. Then cut along the horizontal line so you have a top and bottom scale.

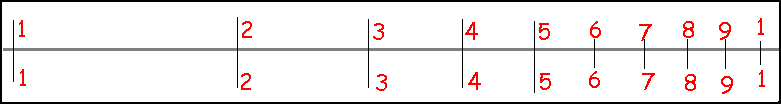
Here is how to multiply 2 by 3: Move the bottom scale to the right so that the 1 on the bottom scale matches the 2 on the top. Now read along the bottom scale to the 3 and see what it says on the top scale. 6! All we have done is added a log of 2 distance on the top to a log of 3 distance on the bottom to get a log of 6 distance on the top. Here is how to divide 9 by three: Move the bottom scale so that the 3 is underneath the 9. Now see what is above the 1 on the bottom scale. 3! So we subtracted distances to divide and add to multiply. What happens if we try to multiply 4 by 5? As for 2 times 3... Put the 1 of the bottom scale against the 4 of the top then read off what is above the 5. Oops. Run off the end of the top scale! Luckily we can use the same trick we used to save printing an enormous book of log tables to save having to make a very long slide rule. So long as we keep track of the tens we can still do all the multiplications and divisions we want then multiply the final result by the tens we took out earlier. Instead of putting the left hand 1 of the bottom scale against the 4 on the top, move the bottom slide to the left so that the right hand 1 is under the 4. Now read off above the 5. You should see 2 which stands for 20. And that is how a slide rule works. What a bore it is when you fall off one end of the scale and have to jump to the other end. Why not tape the ends of each scale together to make two rings and slide them round say a bottle instead of being slid flat on the table.

Tricks from the slide rule era... ...and still useful today

Back of the envelope You might wonder how engineers 'of the old school' managed without modern calculators. Easy: You do rough sums with the figures but make sure you get the decimal point in the right place. For example: If our factory makes 2000 bobbins a week and we have 5 machines, how many is this per year per machine? 2 (000) times 5 (0) divided by 5 which is 2 times 5 divided by 5 = 2 and four 0's so the result is 20,000. With most engineering calculations you leave a Factor of Safety of perhaps four times so quick calculations are often going to be fine.

Reality check How can we check a calculation quickly? One way is to work out the units as a separate sum. Example: How far is the Sun from the Earth? If the speed of light 300000000 metres per second and it takes about 9 minutes for light to reach the Earth then perhaps the answer is 3 times 9 (with 8 zeros on the end) kilometres? Let's see: metres per second is metres divided by seconds. So our sum if worked out in units is: metres times minutes divided by seconds. is this the same as Km? Doesn't look quite right does it. Let's have another go: words units figures Speed of light per second in metres m/s 3 (plus 8 0's) multiplied by number of seconds s 9 times 60=540 5.4 (plus 2 0's) equals number of metres m/s times s = m the s's cancel out... 3 times 5.4 = 16.2 8 0's plus 2 0's = 10 0's adjust into kilometres... 1000 metres per kilometer is 1/1000th km per metre km/m .001 1 ('minus' 3 0's) gives our result kilometers m times km divided by m. Cancelling the m's leaves us with km 16.2 times by 1 is 16.2 10 0's minus 3 0's is 7 0's Result: 162,000,000 km Actually "number of seconds" above is shorthand for "journey time taken for light to reach the Earth from the Sun in seconds." The complete calculation in units is: m s km km See how the s's and m's cancel out. X X = s journey m journey